Best way to solve Mole Calculations using Molar Volume 

In the SQA Higher Chemistry course, students are expected to be able to use molar volume (M_v) and calculate the amount of a gas (litres) given off using mole equations. Using the triangle in the diagram below, the relationships between the number of moles (n), molar (M_v ) and volume (V) can be determine where n = V/M_v and V = n * M_v . The molar volume can be defined as the volume of gas in mole of a substance, with units of mol/l (mole per litre), which in most cases in constant. In many chemical reactions, there is gas given off, such as carbon dioxide (burning of hydrocarbons) and hydrogen (reaction of a metal with an acid produces hydrogen gas). Working out how much gas is given off is possible by using these formulas, which could be applied to a real scenario, such as a chemical plant.

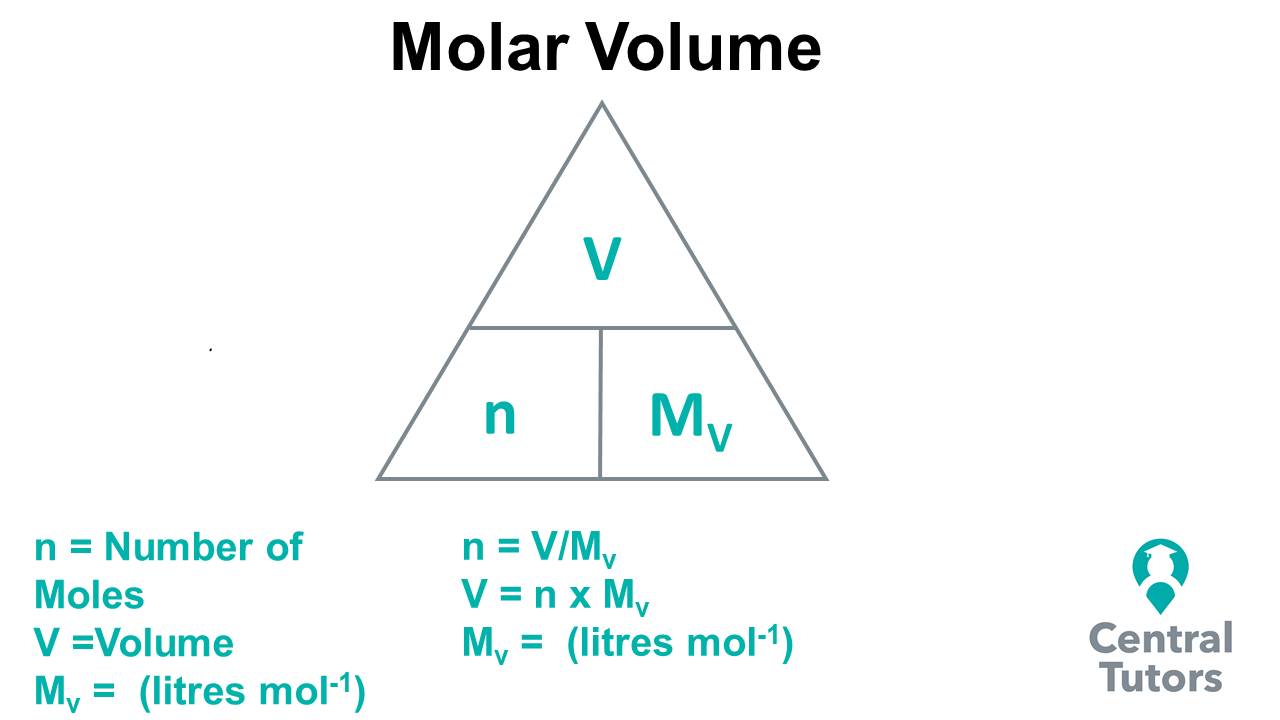
By applying the above formulas, we can solve specific problems, relating to mole calculations involving volume.  An example of how to do this is shown below.

Example 1 – How to solve Mole Calculations using Molar Volume 

In the reaction below, 5 grams of Zinc is reacted with excess hydrochloric acid. What is volume of hydrogen gas given off, if the molar volume of hydrogen is 24 mol/l. Using the mole relationships above, and the balanced equation (below), the volume of hydrogen can be calculated.

The gram formula mass of a compound can be calculated using the data booklet and summing together the masses of all the individual elements. In this case however, we only have Zinc, which has a Gram formula mass of 65.4g.

Therefore, because it is a 1 to 1 mole ratio, 0.08 moles of zinc produce 0.08 moles of hydrogen. Now that we know there is 0.08 moles of hydrogen produced, we can now use this to work out the volume of hydrogen gas given off.

Reacting 5 grams of zinc with excess hydrochloric acid, produces 1.92 litres of hydrogen gas. The mole calculation was quite straightforward, because it was a one-to-one mole ratio.

Example 2: Alternative method on solving Mole Calculations

What mass (grams) of propane is burned to produce 150 cm³ of carbon dioxide, using the following equation below:

Therefore, using the same method as above, the mole ratio between carbon dioxide and propane is 3:1, meaning that when 1 mole of propane is burned completely in oxygen, it produces 3 moles of carbon dioxide. This means that is 150cm³ of carbon dioxide was produced, which is the equivalent to 0.00625 moles, then using the mole ratio, there must have only been 0.0021 moles of propane.

Now that the number of moles of propane has been calculated, the mass of propane can be calculated suing the following formula:

Using the mole ratio and the relationship between molar volume, volume and number of moles, many problems can be solved. Following the simple steps above, it is possible to solve most problems in the SQA Higher Chemistry course. Private chemistry tutors will be able to go through specific examples involving molar volume mole calculations, which will help you consolidate the work.